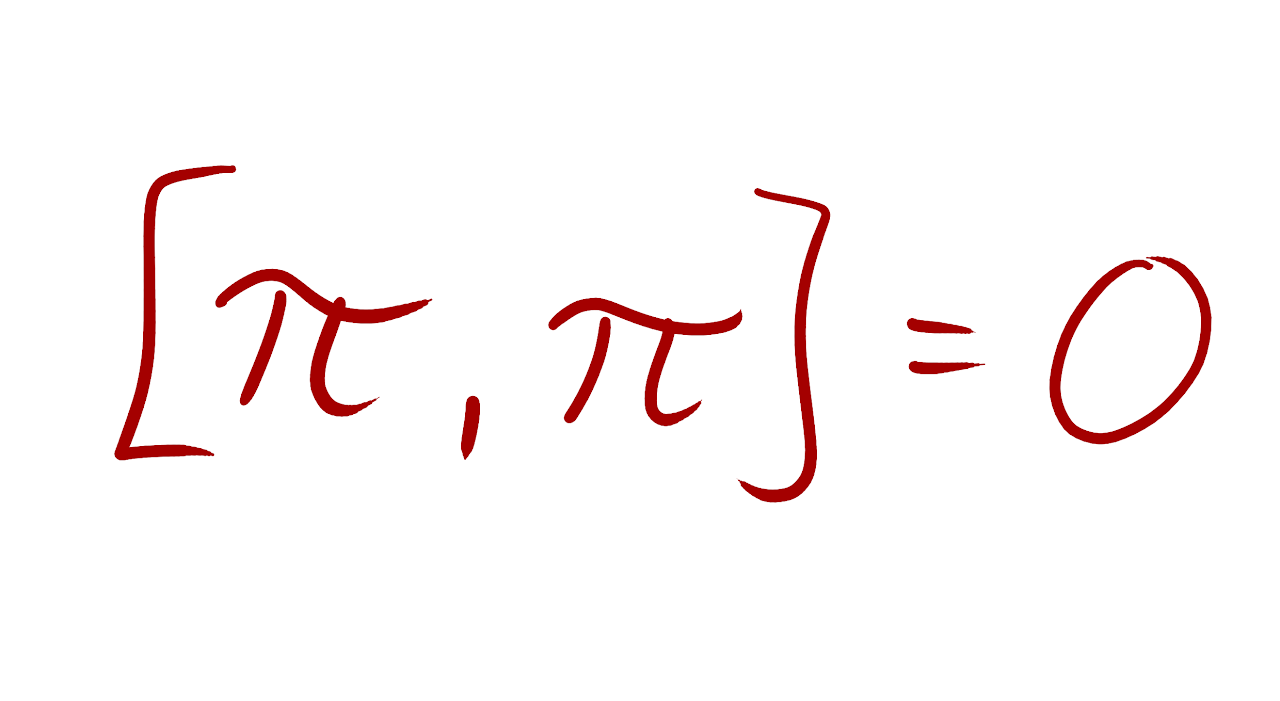Geometry of string theory
Apart from being one of the candidates for the theory of everything, string theory possesses an intriguing mathematical structure and has led to many insights, studies, and discoveries in pure mathematics, for instance in the field of geometry. Part of my research has been focused on unraveling some of the mysterious connections between the two worlds, such as the link between Courant algebroids and string theory.
Exceptional generalised geometry
Exceptional generalised geometry studies the structures arising in M-theory and its compactifications. In particular, it features the famous exceptional Lie groups. Despite its importance and due to its complexity, the theory is much less developed than its more standard stringy "cousin", the generalised geometry. I am currently working on the development of a general framework of the so-called Y-algebroids, which can be used as a bridge to carry some crucial ideas and results from GG to EGG.
Poisson–Lie T-duality and beyond
Dualities provide an invaluable tool in modern physics, displaying links between a priori unrelated physical setups. They are particularly useful and abundant in string theory – for instance, T-duality relates two different toroidal compactifications of the stringy spacetime. In 1995, a vast generalisation of this phenomenon, called Poisson–Lie T-duality, was found by Klimčík and Ševera. I study various aspects of this duality: its relation to sigma models, supergravity, its higher versions and generalisations.
Poisson geometry
Poisson geometry is a geometric study of the structures present in the phase spaces of classical mechanics. In recent decades it has developed into a rich discipline with connections to various other areas, for example the mathematical aspects of quantisation. I have been interested mainly in the study of Courant algebroids — higher cousins of Poisson manifolds.
Graded geometry
It is no secret that many concepts, definitions, and proofs simplify significantly when we look at them from the right angle. In many cases, this right angle seems to be given by what might be called graded geometry – study of spaces whose algebras of functions are ℤ-graded commutative algebras. I am interested in finding graded-geometric reformulations of various ideas, for example those coming from the world of Courant algebroids.